MATHEMAGIC 20: CIRCLES

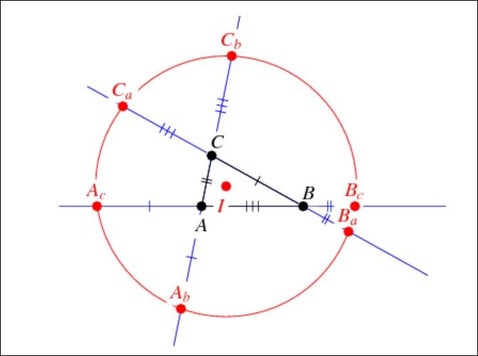
Do you want to know what's the 'Conway circle'?
Go to: http://mathworld.wolfram.com/ConwayCircle.html

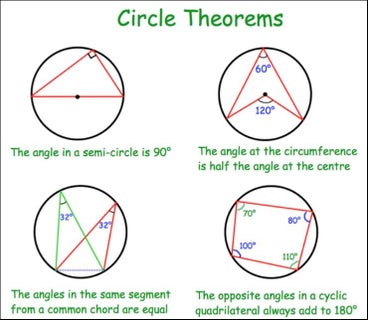
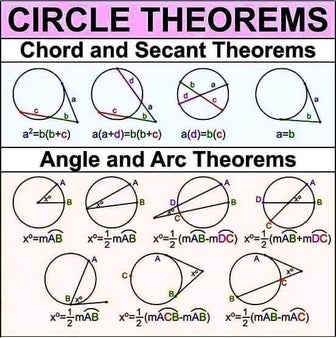
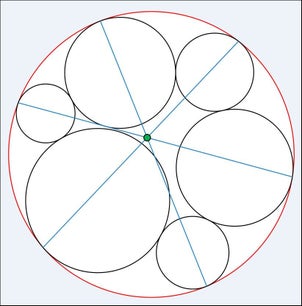
In geometry, the seven circles theorem is a theorem
about a certain arrangement of seven circles in the Euclidean plane.

Specifically, given a chain of six circles all tangent to a seventh circle
and each tangent to its two neighbors, the three lines drawn between opposite pairs of the points of tangency on the seventh circle
all pass through the same point.

Though elementary in nature,
this theorem was not discovered until 1974
(by Evelyn, Money-Coutts, and Tyrrell).

 

From: Wikipedia.

 

I used this logo on my Facebook page.
The picture shows an equilateral triangle
with its inscribed and circumscribed circle.
If the area of the inscribed circle (yellow) equals pi,
than the area of the other three colored pieces also equals pi.

Can you prove it?

 

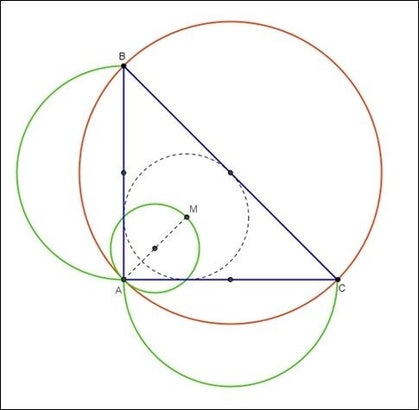
JANUS' CIRCLE THEOREM

Triangle ABC is an equilateral rectangular triangle with hypotenuse [BC].
M is the center of the inscribed circle.
Then the sum of the circumferences of the circles with diameter [AB] and [AM]
equals the circumference of the subscribed circle (with diameter [BC]).

 

GREEN = RED

 

You may find a proof (in Dutch) on

http://blogimages.bloggen.be/gnomon/attach/308668.pdf